Strings

︎
Strings freely interprets the connection between the string theory and Pythagoras’ harmonic theory by observing «microscopic» frequencies and phenomena gained by stimulating an extremely long string.
︎




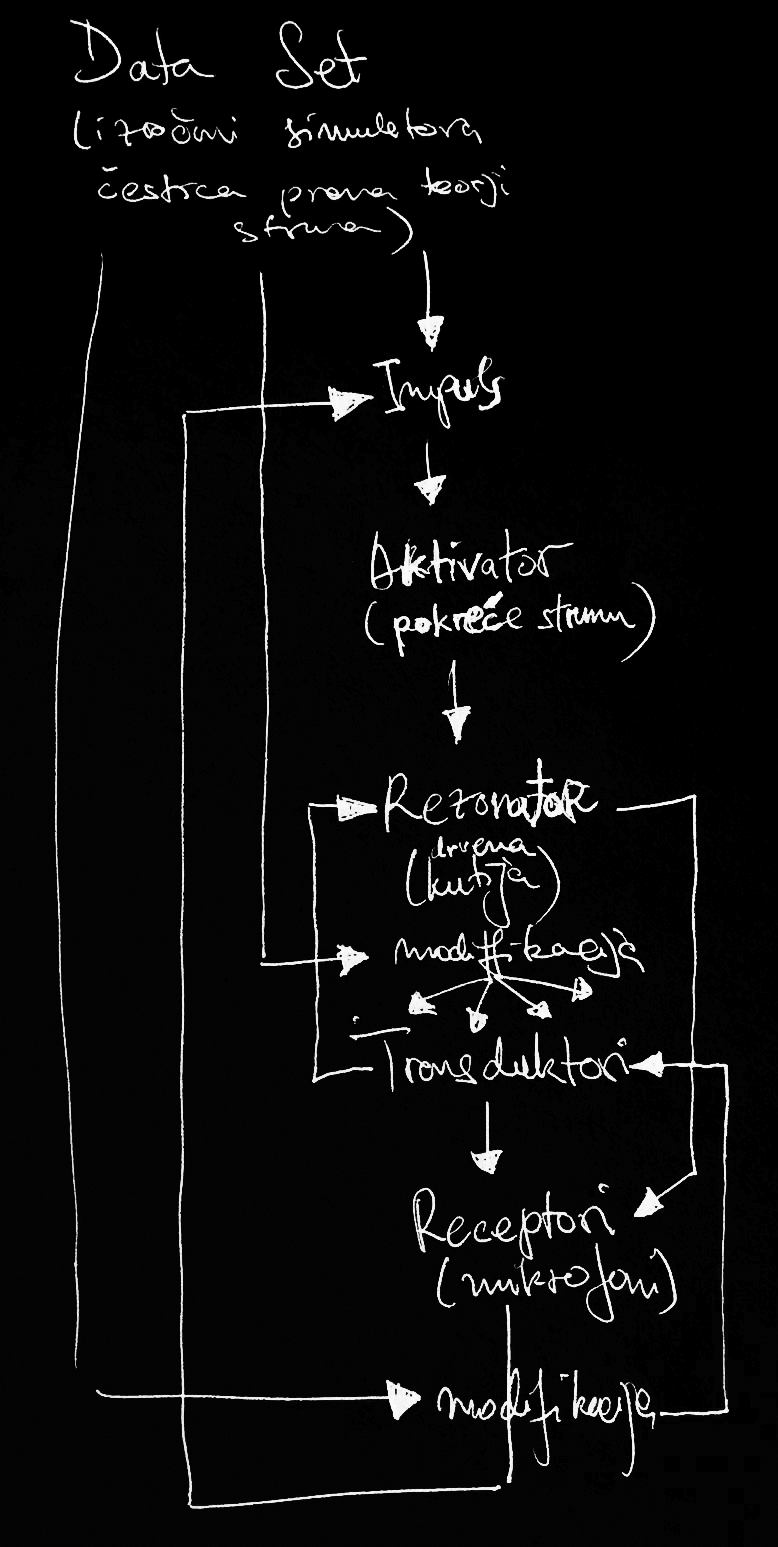
Blueprint of the composition
The data set is obtained from the simulation of the behaviour of the subatomic particles

Exhibition booklet, downladable here ︎
Intro
String is an elastic thread made of metal or artifical fibers, which creates sound by its titration. It's used in chordophone string instruments, e.g. violin, harp or piano. The tone is produced in three ways: by plucking, stroking or with the bow. In theoretical physics string is a hypothetical microscopic structure, by whose titration the particles and the interference are being explained. The predecessor of the string theory is Pythagorean teaching, he was the first to notice the connection between music and mathematics. He discovered harmonics by observing the string movement and that titration of the strings produce tones in harmony when the lenght coefficient of the strings are in whole numbers. He claimed that the music is the language of the universe and that the planets produce celestial music by their interaction - music of the spheres.
The project
Research projest Strings is a collaborative work of the media artist Hrvoje Hiršl and the composer Davor Branimir Vincze. This sound and media research liberally interprets the connection between the string theory and Pythagoras' harmonic theory by observing «microscopic» frequencies and phenomena originating from the sensitization of the extremely long string.
When we say that the sound has the frequency of the middle A, that is 440Hz, we are talking about the frequency of the fundamental tone. Almost every sound found in nature contains lots of other frequencies (harmonics) above the fundamental tone which our ear sums up in one sound. However, small difference in frenquency proportions and intensity of the harmonics makes great differences in the perception which helps with the recognition of different instruments, voices, sound etc. In this work we would like to accent and extract those «hidden» frequencies (harmonics).
The impuls that we send to the sound activator enhances the string vibration. Vibration is then amplified in the resonant box. This simple input sound is then pulled through the computer and by feedback many frequencies which couldn't be heard merely by ear are being extracted. Those frenquencies go through the algorithm in which the sound is modified (transponed, amplified, filtered etc.). The whole algorithm is coordinated via data set we got from our collegues physicists at the Stanford university where they simulate the string theory. The same algorithm is divided into several modules, in which every module has its own type of manipulation of the input sound and therefore different result. In the moment when the specific process gets to its limit, the next module is activated. Enriched sounds by the algorithm are projected into the resonant box.
The data set is obtained by the simulation of the behaviour of the subatomic particles. The string theory says that every matter originates as a vibration in ten dimensions which by their behaviour remind you of the strings. Of these ten dimensions humans percieve three space and one time dimension and the rest are hidden in the subatomic (quantum) level. Those hidden dimensions can't be seen but we can measure the energy generated by the titration in these dimensions. Collision of those atomis particles develops new ones in the higher condition which then fall apart and change direction. The change of direction, that is the position in which the particles are found after falling apart we calculate as a number, which is stored on the list from which the data set is made. Tha data set is used as a measure that modifies the input sound as a part of the electronics. It decides on the amount of the delay, transposition, filtering, amplification etc.
String is an elastic thread made of metal or artifical fibers, which creates sound by its titration. It's used in chordophone string instruments, e.g. violin, harp or piano. The tone is produced in three ways: by plucking, stroking or with the bow. In theoretical physics string is a hypothetical microscopic structure, by whose titration the particles and the interference are being explained. The predecessor of the string theory is Pythagorean teaching, he was the first to notice the connection between music and mathematics. He discovered harmonics by observing the string movement and that titration of the strings produce tones in harmony when the lenght coefficient of the strings are in whole numbers. He claimed that the music is the language of the universe and that the planets produce celestial music by their interaction - music of the spheres.
The project
Research projest Strings is a collaborative work of the media artist Hrvoje Hiršl and the composer Davor Branimir Vincze. This sound and media research liberally interprets the connection between the string theory and Pythagoras' harmonic theory by observing «microscopic» frequencies and phenomena originating from the sensitization of the extremely long string.
When we say that the sound has the frequency of the middle A, that is 440Hz, we are talking about the frequency of the fundamental tone. Almost every sound found in nature contains lots of other frequencies (harmonics) above the fundamental tone which our ear sums up in one sound. However, small difference in frenquency proportions and intensity of the harmonics makes great differences in the perception which helps with the recognition of different instruments, voices, sound etc. In this work we would like to accent and extract those «hidden» frequencies (harmonics).
The impuls that we send to the sound activator enhances the string vibration. Vibration is then amplified in the resonant box. This simple input sound is then pulled through the computer and by feedback many frequencies which couldn't be heard merely by ear are being extracted. Those frenquencies go through the algorithm in which the sound is modified (transponed, amplified, filtered etc.). The whole algorithm is coordinated via data set we got from our collegues physicists at the Stanford university where they simulate the string theory. The same algorithm is divided into several modules, in which every module has its own type of manipulation of the input sound and therefore different result. In the moment when the specific process gets to its limit, the next module is activated. Enriched sounds by the algorithm are projected into the resonant box.
The data set is obtained by the simulation of the behaviour of the subatomic particles. The string theory says that every matter originates as a vibration in ten dimensions which by their behaviour remind you of the strings. Of these ten dimensions humans percieve three space and one time dimension and the rest are hidden in the subatomic (quantum) level. Those hidden dimensions can't be seen but we can measure the energy generated by the titration in these dimensions. Collision of those atomis particles develops new ones in the higher condition which then fall apart and change direction. The change of direction, that is the position in which the particles are found after falling apart we calculate as a number, which is stored on the list from which the data set is made. Tha data set is used as a measure that modifies the input sound as a part of the electronics. It decides on the amount of the delay, transposition, filtering, amplification etc.

We thank dr. Brian Shuve Assistant Professor of Physics Harvey Mudd College
on the given data set.
Special thanks to professor Jonathan Abelu for the help on the acoustis issues.
Photo by Damir Žižić
Special thanks to professor Jonathan Abelu for the help on the acoustis issues.
Photo by Damir Žižić